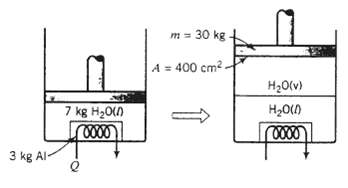
A perfectly insulated cylinder fitted with a leak proof friction less piston with a mass of 30.0 kg and a face area of 400.0 cm² contains 7.0 kg of liquid water and a 3.0-kg bar o1 aluminum. The aluminum bar has an electrical coil imbedded in it, so that known amounts of heat can be transferred to it. Aluminum has a specific gravity of 2.70 and a specific internal energy given by the formula U (kJ/kg) = 0.94T (?C). The internal energy of liquid water at any temperature may be taken to be that of the saturated liquid at that temperature. Negligible heat is transferred to the cylinder wall. Atmospheric pressure is 1.00 atm. The cylinder and its contents are initially at 20?C.

Suppose that 3310 kJ is transferred to the bar from the heating coil and the contents of the cylinder are then allowed to equilibrate.

(a) Calculate the pressure of the cylinder contents throughout the process. Then determine whether the amount of heat transferred to the system is sufficient to vaporize any of the water.

(b) Determine the following quantities: (i) the final system temperature; (ii) the volumes (cm³) of the liquid and vapor phases present at equilibrium; and (iii) the vertical distance traveled by the piston from the beginning to the end of the process. [Suggestion: Write an energy balance on the complete process. taking the cylinder contents to be the system. Note that the system is closed and that work is done by the system when it moves the piston through a vertical displacement. The magnitude of this work is W = P?V, where P is the constant system pressure and V is the change in system volume from the initial to the final state.]

(c) Calculate an upper limit on the temperature attainable by the aluminum bar during the process and state the condition that would have to apply for the bar to come close to this temperature.

Transcribed Image Text:

3 kg Al- 7 kg H₂O( 0000 m = 30 kg. A= 400 cm². H₂O(v) H₂O(n) 0000