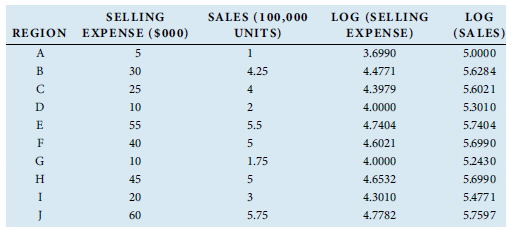
A product manager has been reviewing selling expenses (that is, advertising, sales commissions, and so on) associated with marketing a line of household cleaning products. The manager suspects that there may be some sort of diminishing marginal returns relationship between selling expenses and the resulting sales generated by these expenditures. After examining the selling expense and sales data for various regions (all regions are similar in sales potential) shown in the following table and graph, however, the manager is uncertain about the nature of the relationship.

a. Using the linear regression model

Y = α + βX

Where Y is sales and X is selling expenses, estimate α, β, and the r2 statistic by the least-squares technique.

b. Using the exponential function model

Y = αXβ

Apply the double-logarithmic transformation to obtain a linear relationship that can be estimated by the least-squares technique.

c. Applying the least-squares technique, estimate α, β, and the r2 statistic for the transformed (linear) model in part (b). (The logarithms of the X and Y variables needed in the calculations are given in the table.)

d. Based on the r2 statistics calculated in parts (a) and (c), which model appears to give a better fit of the data?

e. What implications does the result in part (d) have for the possible existence of a diminishing marginal returns relationship between sales and selling expenses as suggested by the manager?

f. What other transformations of the variables might we try to give a better fit to the data?

Transcribed Image Text:

LOG (SELLING EXPENSE) SELLING SALES (100,000 UNITS) LOG REGION EXPENSE ($000) (SA LES) 5 3.6990 5.0000 30 4.25 4.4771 5.6284 25 4.3979 5.6021 D 10 2 4.0000 5.3010 55 5.5 4.7404 5.7404 40 4.6021 5.6990 G 10 1.75 4.0000 5.2430 Н 45 4.6532 5.6990 20 3 4.3010 5.4771 60 5.75 4.7782 5.7597