A charged particle, traveling in from ?? ?? along the x axis, encounters a rectangular potential energy
Question:
A charged particle, traveling in from ?? ?? along the x axis, encounters a rectangular potential energy barrier Show that, because of the radiation reaction, it is possible for the particle to tunnel through the barrier--that is: even if the incident kinetic energy is less than U0, the particle can pass through. (See F. Denef et al., Phys. Rev. E 56, 3624 (1997).) Refer to Probs. 11.19 and 11.28, but notice that this time the force is a specified function of x, not t. Them are three regions to consider: (i) x L. Find the general solution (for a(t), v(t), and x(t)) in each region, exclude the runaway in region (iii), and impose the appropriate boundary conditions at x = 0 and x = L. Show that the final velocity (v f) is related to the time T spent traversing the barrier by the equation and the initial velocity (at x = ?? ??) is To simplify these results (since all we're looking for is a specific example), suppose the final kinetic energy is half the barrier height. Show that in this case In particular, if you choose L = vfτ/4, then vi = (4/3)vf, the initial kinetic energy is (8/9)U0, and the particle makes it through, even though it didn't have sufficient energy to get over the barrier!]
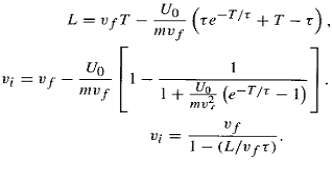
Step by Step Answer:






