Consider a linear time-invariant system whose impulse response is real and is given by h[n]. Suppose the responses of the system to the two inputs x[n] and v[n] are, respectively, y[n] and z[n], as shown in Figure. The inputs x[n] and v[n] in the figure represent real zero-mean stationary random processes with auto correlation functions ?_xx[n] and ?_vv[n], cross-correlation function ?_xv[n], power spectra ?_xx(e^j?) and ?_vv(e^j?), and cross power spectrum ?_xv(e^j?).

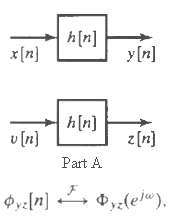
(a) Given ?_xx[n],?_vv[n], ?_xv[n],?_xx(e^j?), ?_vv(e^j?), and ?_xv(e^j?), determine ?_yz(e^j?), the cross power spectrum of y[n] and z[n], where ?_yz(e^j?) is defined by

?with ?_yz[n] = E{y[k]z[k ? n]}.

(b) Is the cross power spectrum ?_xv(e^j?) always nonnegative; i.e., is ?_xv(e^j?) ? 0 for all ?? Justify your answer.

h[n] x[n] y[n] h[n] v [n] z[n] Part A