Dimensionless variables for free convection, the dimensionless variables in Eqs 11.4-39 to 43 can be obtained by
Question:
Dimensionless variables for free convection, the dimensionless variables in Eqs 11.4-39 to 43 can be obtained by simple arguments. The form of Θ is dictated by the boundary conditions and that of ζ is suggested by the geometry. The remaining dimensionless variables may be found as follows:
(a) Set η = y/y0, Φ2 = v2/vz0, and Φy = vy/vy0, the subscript-zero quantities being constants, then the differential equations in Eqs. 11.4-33 to 35 become with the boundary conditions given in Eqs. 11.4-47 to 49.
(b) Choose appropriate values of vz0, vy0, and y0 to convert the equations in (a) into Eqs. 11.4-44 to 46, and show that the definitions in Eqs. 11.4-41 to 43 follow directly.
(c) Why is the choice of variables developed in (b) preferable to that obtained by setting the three dimensionless groups in Eqs, 11B.15-1 and 2 equal to unity?
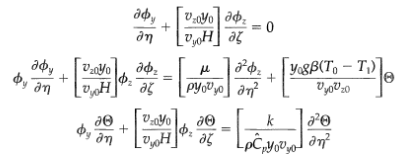
Step by Step Answer:






