Electrons undergoing cyclotron motion can be speeded up by increasing the magnetic field; the accompanying electric field

Question:

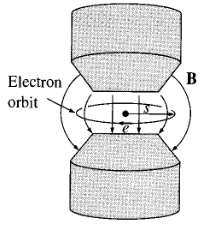
Electrons undergoing cyclotron motion can be speeded up by increasing the magnetic field; the accompanying electric field will impart tangential acceleration. This is the principle of the betatron. One would like to keep the radius of the orbit constant during the process. Show that this can be achieved by designing a magnet such that the average field over the area of the orbit is twice the field at the circumference (Fig. 7.52). Assume the electrons start from rest in zero field and that the apparatus is symmetric about the center of the orbit. (Assume also that the electron velocity remains well below the speed of light, so that non relativistic mechanics applies.)