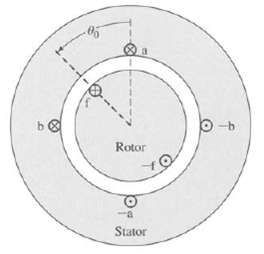
Figure shows in cross section a machine having a rotor winding f and two identical stator windings a and b whose axes are in quadrature. The self-inductance of each stator winding is L_aa and of the rotor is L_ff. The air gap is uniform. The mutual inductance between a stator winding depends on the angular position of the rotor and may be assumed to be of the form M_af = M cos ?₀ M_bf = M sin ?_0?where M is the maximum value of the mutual inductance. The resistance of each stator winding is Ra.

a. Derive a general expression for the torque T in terms of the angle ?₀, the inductance parameters, and the instantaneous currents i_a, I_b, and i_f. Does this expression apply at standstill? When the rotor is revolving?

b. Suppose the rotor is stationary and constant direct currents l_a = I₀, I_b = I₀, and I_f = 21₀ are supplied to the windings in the directions indicated by the dots and crosses in figure. If the rotor is allowed to move, will it rotate continuously or will it tend to come to rest, if the latter at what value of ?₀?

c. The rotor winding is now excited by a constant direct current If while the stator windings carry balanced two-phase currents i_a = ?2I_a cos wt i_b = ?2I_a sin wt The rotor is revolving at synchronous speed so that its instantaneous angular position is given by ?₀ = wt = ?, where ? is a phase angle describing the position of the rotor at t = 0. The machine is an elementary two-phase synchronous machine. Derive an expression for the torque under these conditions.

d. Under the conditions of part (c), derive an expression for the instantaneous terminal voltages of stator phases a and b.

Transcribed Image Text:

ье O-b Rotor Stator