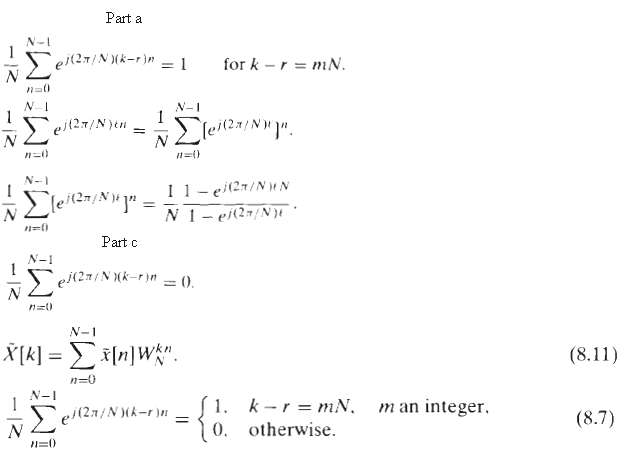
In deriving the DFS analysis equation (8.11), we used the identity of Eq. (8.7). To verify this identity, we will consider the two conditions k ? r = m N and k ? r ? m N separately.

(a) For k ? r = m N, show that e ^{j (2?/N) (k ? r) n} = 1 and, from this, that since k and r are both integers in Eq. (8.7), we can make the substitution k ? r = ? and consider the summation, because this is the sum of a finite number of terms in a geometric series, it can be expressed in closed from as

(b) For what values of ? is the right-hand side of this equation indeterminate? That is, are the numerator and denominator both zero?

(c) From the result in part (b), show that if k ? r ? m N, then?

Part a Teil27/Nk-r)n = 1 for k -r = mN. %3D leil2a/NW". , n=) I 1- e(27/NH N N1-ei(2/N %3! Part c N-1 I5 ei(27/N 1(k-r)m =0. %3D N-1 X|k] = F ]W*". (8.11) n=0 N-1 S1. k-r mN. man integer, 0. otherwise. e(27/N XA-r)n (8.7) l=0 -12 -12