In this problem you will derive Equation 35-28 for the resolving power of a diffraction grating containing
Question:
In this problem you will derive Equation 35-28 for the resolving power of a diffraction grating containing N slits separated by a distance d. To do this you will calculate the angular separation between the maximum and minimum for some wavelength λ and set it equal to the angular separation of the mth-order maximum for two nearby wavelengths.
(a) Show that the phase difference ф between the light from two adjacent slits is given by
-1.png)
(b) Differentiate this expression to show that a small change in angle dθ results in a change in phase of dф given by
-2.png)
(c) For N slits, the angular separation between an interference maximum and interference minimum corresponds to a phase change of dф = 2π/N. Use this to show that the angular separation dθ between the maximum and minimum for some wavelength λ is given by
-3.png)
(d) The angle of the mth-order interference maximum for wavelength λ is given by Equation 35-27. Compute the differential of each side of this equation to show that angular separation of the mth-order maximum for two nearly equal wavelengths differing by dλ is given by
-4.png)
(e) According to Rayleigh’s criterion, two wavelengths will be resolved in the mth order if the angular separation of the wavelengths given by Equation 35-31 equals the angular separation of the interference maximum and interference minimum given by Equation 35-30. Use this to derive Equation 35-28 for the resolving power of a grating.
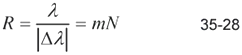
Step by Step Answer:

Fundamentals of Ethics for Scientists and Engineers
ISBN: 978-0195134889
1st Edition
Authors: Edmund G. Seebauer, Robert L. Barry





