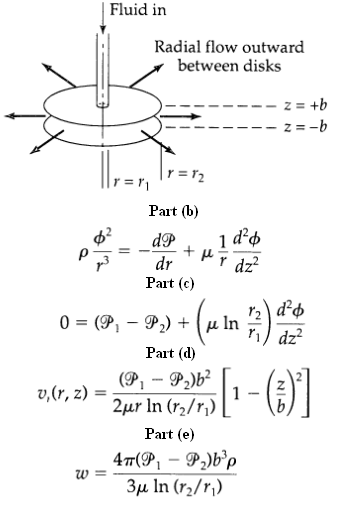
Radial flow between parallel disks (Fig. 3B.10). A part of a lubrication system consists of two circular disks between which a lubricant flows radially. The flow takes place because of a modified pressure difference P₁ – P₂ between the inner and outer radii r₁ and r₂, respectively. 

(a) Write the equations of continuity and motion for this flow system, assuming steady-state, laminar, incompressible Newtonian flow. Consider only the region r₁ < r < r₂ and a flow that is radially directed. Fig. 3B.10 outward radial flow in the space between two parallel, circular disks 

(b) Show how the equation of continuity enables one to simplify the equation of motion to give in which Ф = rv, is a function of z only. Why is Ф independent of r? 

(c) It can be shown that no solution exists for Eq. 3B.10-1 unless the nonlinear term containing & is omitted. Omission of this term corresponds to the "creeping flow assumption." Show that for creeping flow, Eq. 3B.10-1 can be integrated with respect to r to give 

(d) Show that further integration with respect to z gives 

(e) Show that the mass flow rate is 

Fluid in Radial flow outward between disks z = +b z = -b r = r2 Part (b) 1 do dz? dP dr Part (c) r2) do dz? 0 = (P, P2) + (u In Part (d) (P, - P2)b? 2r In (r2/r,) v,(r, z) = Part (e) 47(P, - P2)bp 3 In (r2/r,)