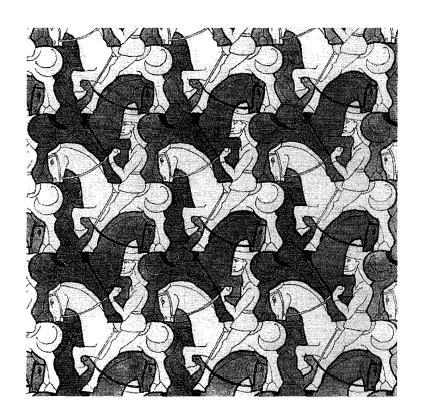
Illustrate the seven different types of friezes when they are classified according to their symmetries. Imagine the
Question:
Illustrate the seven different types of friezes when they are classified according to their symmetries. Imagine the figure shown to be continued infinitely to the right and left. The symmetry group of a frieze always contains translations. For each of these exercises answer these questions about the symmetry group of the frieze.
a. Does the group contain a rotation?
b. Does the group contain a reflection across a horizontal line?
c. Does the group contain a reflection across a vertical line?
d. Does the group contain a nontrivial glide reflection?
e. To which of the possible groups Z, D∞ , Z x Z2, or D∞ x Z2 do you think the symmetry group of the frieze is isomorphic?
H H H H H H H H H H
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
a Yes ...View the full answer
Answered By
Mehwish Aziz
What I have learnt in my 8 years experience of tutoring is that you really need to have a friendly relationship with your students so they can come to you with their queries without any hesitation. I am quite hardworking and I have strong work ethics. Since I had never been one of those who always top in the class and always get A* no matter what, I can understand the fear of failure and can relate with my students at so many levels. I had always been one of those who had to work really hard to get decent grades. I am forever grateful to some of the amazing teachers that I have had who made learning one, and owing to whom I was able to get some extraordinary grades and get into one of the most prestigious universities of the country. Inspired by those same teachers, I am to be like one of them - who never gives up on her students and always believe in them!
3+ Reviews
10+ Question Solved
Related Book For 
Question Posted:
