Let F be any field. Consider the system of m simultaneous linear equations inn unknowns where a
Question:
Let F be any field. Consider the "system of m simultaneous linear equations inn unknowns"
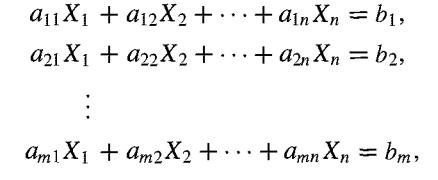
where aij, bi ∈ F.
a. Show that the "system has a solution;' that is, there exist X1, ··· , Xn ∈ F that satisfy all m equations, if and only if the vector β = (b1 , ··· , bm) of Fm is a linear combination of the vectors αj = ( a1j , ··· , amj). (This result is straightforward to prove, being practically the definition of a solution, but should really be regarded as the fundamental existence theorem for a simultaneous solution of a system of linear equations.)
b. From part (a), show that if n = m and {αj |j = 1, ··· , n} is a basis for Fn, then the system always has a unique solution.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





