By the principles used in modeling the string it can be shown that small free vertical vibrations
Question:
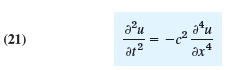
where c2 = EI/ÏA (E = Young€™s modulus of elasticity, I = moment of intertia of the cross section with respect to the y-axis in the figure, Ï = density, A = cross-sectional area).
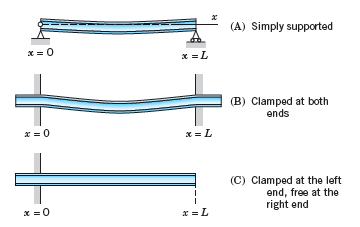
If the beam is clamped at the left and free at the right (Fig. 293C), the boundary conditions are
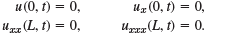
Show that F in Prob. 15 satisfies these conditions if βL is a solution of the equation
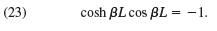
Find approximate solutions of (23).
Data from Prob. 15
By the principles used in modeling the string it can be shown that small free vertical vibrations of a uniform elastic beam (Fig. 292) are modeled by the fourth-order PDE
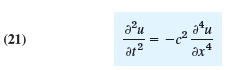
where c2 = EI/ÏA (E = Young€™s modulus of elasticity, I = moment of intertia of the cross section with respect to the y-axis in the figure, Ï = density, A = cross-sectional area).
Substituting u = F(x)G(t) into (21), show that
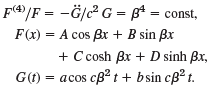
Step by Step Answer:






