Following function is important in applied mathematics and physics (probability theory and statistics, thermodynamics, etc.) and fits our present discussion.

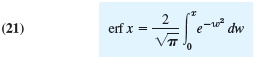
Regarding it as a typical case of a special function defined by an integral that cannot be evaluated as in elementary calculus, do the following.

Obtain the values required in Prob. 10 by an integration command of your CAS. Compare accuracy.

Data from Prob. 10

Following function is important in applied mathematics and physics (probability theory and statistics, thermodynamics, etc.) and fits our present discussion.

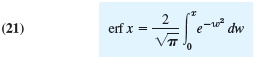
Regarding it as a typical case of a special function defined by an integral that cannot be evaluated as in elementary calculus, do the following.

Obtain the Maclaurin series of erf x from that of the integrand. Use that series to compute a table of erf x for x = 0(0.01)3 (meaning x = 0, 0.01, 0.02, · · ·, 3).