Show that the shortest curve that has an area (A) below it is a circular arc, [

Question:

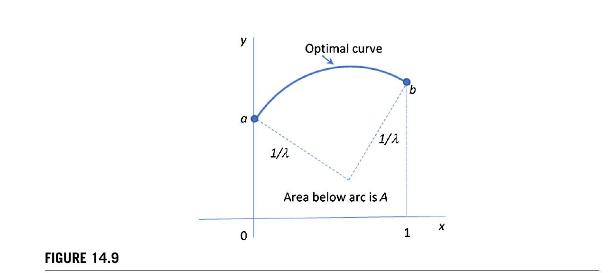
Show that the shortest curve that has an area $A$ below it is a circular arc,
\[
(\lambda x-c)^{2}+(\lambda y-d)^{2}=1,
\]
as shown in Fig. 14.9. Here $\lambda$ is a Lagrange multiplier constant and $c$ and $d$ are constants of integration. For this constrained problem, $y(0)=a, y(1)=b$, and $A=\int_{0}^{1} y(x) d x$.

FIGURE 14.9 y a 0 1/2 Optimal curve 1/2 Area below arc is A b X