Calculate the ignition delay period (in deg ca) in a diesel engine using Eqns (16.15a) and (16.15b)
Question:
Calculate the ignition delay period (in deg ca) in a diesel engine using Eqns (16.15a) and (16.15b) using the conditions at the beginning of fuel injection that exist after compression in the engine with the following initial parameters.
Compare the results to those calculated by the equation proposed by Watson (1979):
\[t_{\mathrm{ig}}=\frac{3.52 \mathrm{e}^{\left(2100 / T_{\mathrm{ig}}\right)}}{p_{\mathrm{ig}}^{1.022}}\]
Conditions at ivc: \(p=1.3 \mathrm{bar}\); \(T=310 \mathrm{~K}\); compression index, \(n_{\mathrm{c}}=1.35\). Assume \(\mathrm{CN}=50\).
(a) Effective compression ratio, \(r=10: 1\); engine speed, \(N=2000 \mathrm{rev} / \mathrm{min}\).
(b) Effective compression ratio, \(r=15: 1\); engine speed, \(N=2000\) and \(4000 \mathrm{rev} / \mathrm{min}\).
Comment on these results - do they seem reasonable?
[
(a) \(78.7 ; 27.8 ; 5.61 ;\)
(b) \(16.92 ; 10.63 ; 2.57 ; 33.84 ; 21.27 ; 4.59]\)
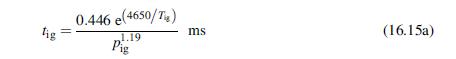
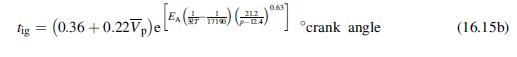
Step by Step Answer:

Advanced Thermodynamics For Engineers
ISBN: 9780080999838
2nd Edition
Authors: D. E. Winterbone, Ali Turan





