The icosahedral group I (Schnflies) or 532 is large, with 60 members and five irreducible representations of

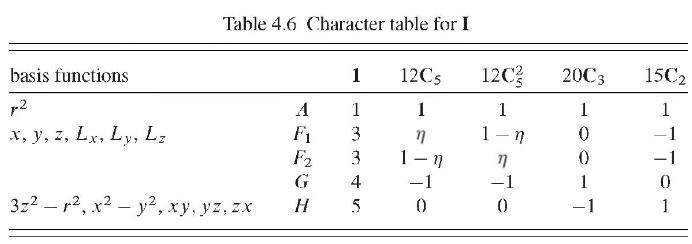
The icosahedral group I (Schönflies) or 532 is large, with 60 members and five irreducible representations of orders \(A=1, F_{1}=3, F_{2}=3, G=4\), and \(H=5\). Table 4.6 shows its character table (omitting inversion) and where \(\eta=\frac{1+\sqrt{5}}{2}\).

(a) Why is I not a crystallographic point group?

(b) For the group \(\mathbf{I}_{h}=\mathbf{I} \otimes \mathbf{i}\) what are the basis functions for gerade (+) and ungerade \((-\) ) representations?

Transcribed Image Text:

Table 4.6 Character table for I basis functions 1 12Cs 12C2 20C3 15C2 F-2 A 1 1 1 1 1 x, y, z, Lx, Ly, Lz F1 3 n 1 0 -1 F2 3 - n n 0 -1 G 4 -1 -1 1 0 - 3z2 r2, x2 y2, xy, yz, zx H 5 0 0 T 1 1