True to the motto “just in time”—the principle of flexible and quick response—a car parts supplier keeps only a small number of injection pumps in stock. Within an hour of receiving an order by telephone, the supplier delivers the injection pump to the car repair shop. The delivery time x fluctuates according to the density function: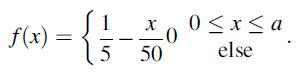

(a) How large is a?

(b) Draw the density function.

(c) Determine using the density function.

(d) What is the associated distribution function?

(e) Draw the distribution function.

(f) Calculate the probability from (c) using the distribution function.

(g) Show the relationship between density and distribution function using your drawings.

(h) Calculate and interpret the percentiles x_0.25, x_0.5, and x_0.75 as well as the quartile range.

(i) How can these values be calculated graphically?

(j) What are the expected value, variance, and standard deviation of the delivery time?

Transcribed Image Text:

f(x) { 5 -0 50 0≤x≤ a else