Consider the following two bimatrix games (i) and (ii): For each bimatrix game, do the following: (a)
Question:
Consider the following two bimatrix games (i) and (ii):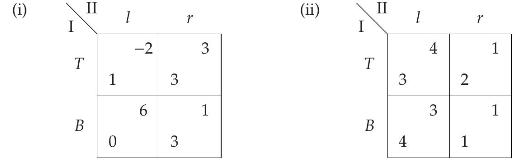
For each bimatrix game, do the following:
(a) Find max-min strategies \(\hat{x}\) and \(\hat{y}\) (which may be mixed strategies) and corresponding max-min values \(u_{0}\) and \(v_{0}\) for player I and II, respectively.
(b) Draw the convex hull of the payoff pairs in the \(2 \times 2\) game, and the bargaining set \(S\) that results from the payoff pairs \((u, v)\) with \(u \geq u_{0}\) and \(v \geq v_{0}\) with the threat point \(\left(u_{0}, v_{0}\right)\) found in (a). Indicate the Pareto-frontier of \(S\).
(c) Find the Nash bargaining solution \((U, V)\).
(d) Show how the players can implement the Nash bargaining solution for \(S\) with a joint lottery over strategy pairs in the \(2 \times 2\) game.
Step by Step Answer:






