Consider the following particular case of the two way layout. Suppose that eight plots are harvested on four of which one variety has been sown, while a different variety has been sown on the other four. Of the four plots with each variety, two different fertilizers have been used on two each. The yield will be normally distributed with a mean θ dependent on the fertiliser and the variety and with variance ϕ. It is supposed a priori that the mean for plots yields sown with the two different varieties are independently normally distributed with mean a and variance ψ_α, while the effect of the two different fertilizers will add an amount which is independently normally distributed with mean β and variance ψ_β. This fits into the situation described in Section 8.6 with ф being ∅ times an 8 x 8 identity matrix and 

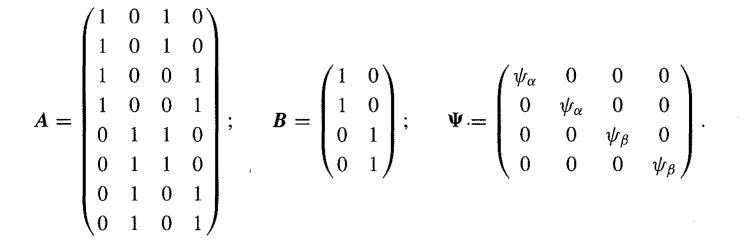
Find the matrix K^-1 needed to find the posterior of θ.

Transcribed Image Text:

A = 1 0 10 1 0 1 0 1 0 0 1 1 0 0 1 0 1 1 0 0 1 1 0 0 1 0 1 0 1 01 ; B = 1 0 0 0 0 1 V. Va 00 0 0 0 Va 0 0 0 0 0 0 VB 0 VB