Suppose that, in a Markov chain with just two states, the probabilities of going from state i to state j in one time unit are given by the entries of the matrix in which i represents the row and j the column Show that the probability of getting from state i to state j in t time units is given by th...

Certainly The probability of transitioning from state i to state j in t time units in a Markov chain ...

Suppose that, in a Markov chain with just two states, the probabilities of going from state i

Question:

Suppose that, in a Markov chain with just two states, the probabilities of going from state i to state j in one time unit are given by the entries of the matrix

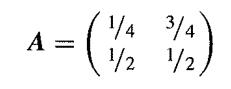
A - (1/2 1/4 3/4 1/2 1/2)

in which i represents the row and j the column. Show that the probability of getting from state i to state j in t time units is given by the tth power of the matrix A and that

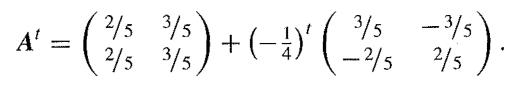
( * ), (-) + ( $/ $ / 2 ) 3) + (- 9)' ( 215 - 2/5 3/5 A

Deduce that, irrespective of the state the chain started in, after a long time it will be in the first state with probability 2/s and in the second state with probability 3/5.

Fantastic news! We've Found the answer you've been seeking!