The Helmert transformation is defined by the matrix so that the element a ij in row i,
Question:
The Helmert transformation is defined by the matrix
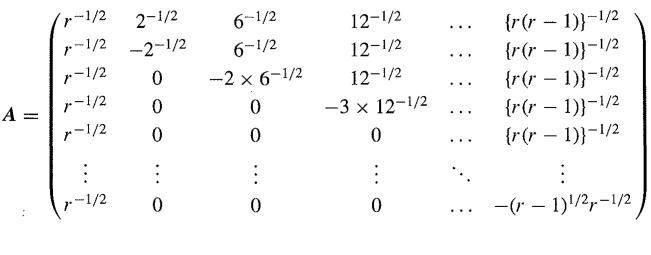
so that the element aij in row i, column j is
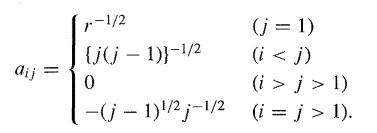
It is also useful to write aj for the ( column) vector which consists of the jth column of the matrix A. Show that if the variates Xi are independently N(θi,1), then the variates Wj = ajT /(X - µ) = ∑i ai j (Xi - µj) are independently normally distributed with unit variance and such that EWj = 0 for j > 1 and 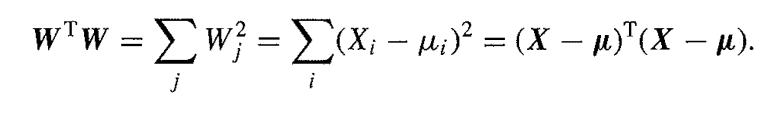
By taking aiJ ∝ θj - µj for i > j, ajj = 0 for i jj such tha ∑j aij = 0, extend this result to the general case and show that E W1 ∝ y = ∑i (0i - µi)2. Deduce that the distribution of a non-central chi-squared variate depends only of r and y.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





