Figure 14 shows the graph of (x) = x sin x. Let (a) Locate the local max
Question:
Figure 14 shows the graph of ƒ(x) = x sin x. Let 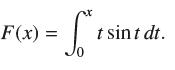
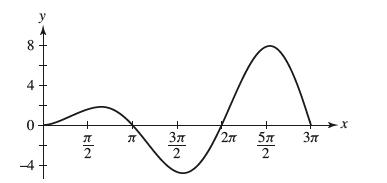
(a) Locate the local max and absolute max of F on [0, 3π].
(b) Justify graphically: F has precisely one zero in [π, 2π].
(c) How many zeros does F have in [0, 3π]?
(d) Find the inflection points of F on [0, 3π]. For each one, state whether the concavity changes from up to down or from down to up.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





