Let (mathbf{F}) be a vector field whose curl and divergence at the origin are [ operatorname{curl}(mathbf{F})(0,0,0)=langle 2,-1,4angle,

Question:

Let $\mathbf{F}$ be a vector field whose curl and divergence at the origin are
\[
\operatorname{curl}(\mathbf{F})(0,0,0)=\langle 2,-1,4angle, \quad \operatorname{div}(\mathbf{F})(0,0,0)=-2
\]

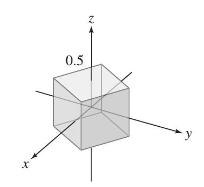
Estimate the flux of $\mathbf{F}$ through the box of side 0.5 in Figure 4 . Does the result depend on how the box is oriented relative to the coordinate axes?

image text in transcribed