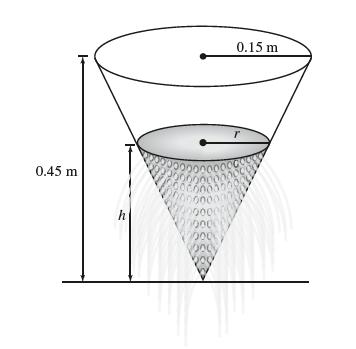
The conical watering pail in Figure 18 has a grid of holes. Water flows out through the holes at a rate of kA m³/min, where k is a constant and A is the surface area of the part of the cone in contact with the water. This surface area is A = πr √h² + r² and the volume is V = 1/3 πr²h. Calculate the rate dh/dt at which the water level changes at h = 0.3 m, assuming that k = 0.25 m.

Transcribed Image Text:

Roy 0.15 m (500) DOLC ნილოს. 0000 00000000 1000 0 0 0 0 0 200 200 0000000 h 0.45 m |-