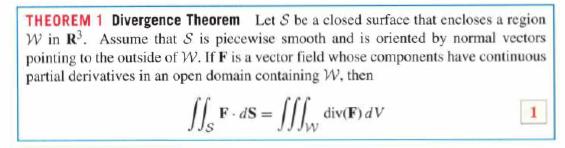
Use the Divergence Theorem to calculate \(\iint_{\mathcal{S}} \mathbf{F} \cdot d \mathbf{S}\) for the given vector field and surface.

\(\mathbf{F}(x, y, z)=\left\langle x y z+x y, \frac{1}{2} y^{2}(1-z)+e^{x}, e^{x^{2}+y^{2}}ightangle, \mathcal{S}\) is the boundary of the solid bounded by the cylinder \(x^{2}+y^{2}=16\) and the planes \(z=0\) and \(z=y-4\).

THEOREM 1 Divergence Theorem Let S be a closed surface that encloses a region W in R. Assume that S is piecewise smooth and is oriented by normal vectors pointing to the outside of W. If F is a vector field whose components have continuous partial derivatives in an open domain containing W, then 1 - Sw div(F) dv F-dS=