Verify Greens Theorem by using a computer algebra system to evaluate both the line integral and the double integral P(x, y) y 2 e x , Q(x, y) x 2 e y , C consists of the line segment from (1, 1) to (1, 1) followed by the arc of the parabola y 2 x 2 from (1, 1) to (1, 1) Data from Green 's Theorem L...

To verify Greens Theorem using a computer algebra system we can evaluate both the line integral and ...

Verify Greens Theorem by using a computer algebra system to evaluate both the line integral and the

Question:

Verify Green’s Theorem by using a computer algebra system to evaluate both the line integral and the double integral.

P(x, y) = y²e^x, Q(x, y) = x²e^y, C consists of the line segment from (–1, 1) to (1, 1) followed by the arc of the parabola y = 2 – x² from (1, 1) to (–1, 1)

Data from Green's Theorem

Let C be a positively oriented, piecewise-smooth, simple closed curve in the plane and let D be the region bounded by C. If P and Q have continuous partial derivatives on an open region that contains D, then

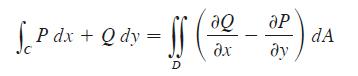
If | Pdx + Q dy = D dA