Johann Bernoulli (1667–1748) evaluated the arc length of curves of the form y = ^{x(2n + 1)/2n}, where n is a positive integer, on the interval [0, a].

a. Write the arc length integral.

b. Make the change of variables 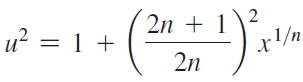to obtain a new integral with respect to u.

c. Use the Binomial Theorem to expand this integrand and evaluate the integral.

d. The case n = 1 (y = x^3/2) was done in Example 1. With a = 1, compute the arc length in the cases n = 2 and n = 3. Does the arc length increase or decrease with n?

e. Graph the arc length of the curves for a = 1 as a function of n.

Transcribed Image Text:

Н): 2n + 1\² 2n r1/n u? = 1 +