Let f(x) sin x and let p n and q n be nth order Taylor polynomials for f centered at 0 and , respectively a Find p 5 and q 5 b Graph f, p 5 , and q 5 on the interval , 2 On what interval is p 5 a better approximation to f than q 5 On what interval is q 5 a better approximation to f than p 5 c Compl...

a We have Thus f0 f4 0 sin 0 0 f0 f5 0 cos 0 1 sin 00 f 0 f 0 cos 0 1 Px c Evaluating the ...

Let f(x) = sin x and let p n and q n be nth order Taylor polynomials

Question:

Let f(x) = sin x and let p_n and q_n be nth order Taylor polynomials for f centered at 0 and π, respectively.

a. Find p₅ and q₅.

b. Graph f, p₅, and q₅ on the interval [-π, 2π]. On what interval is p₅ a better approximation to f than q₅? On what interval is q₅ a better approximation to f than p₅?

c. Complete the following table showing the errors in the approximations given by p₅and q₅ at selected points.

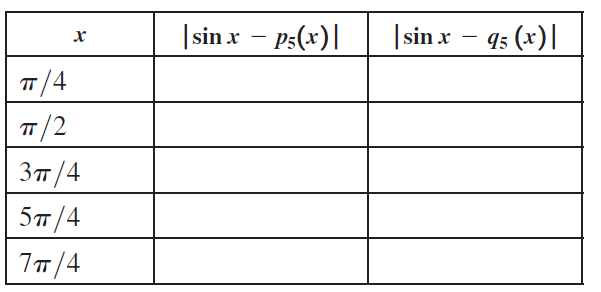
|sin x – ps(x)| |sin x – 45 (x)| х TT/4 T/2 Зп /4 5/4 7п /4

d. At which points in the table is p₅ a better approximation to f than q₅? At which points do p₅ and q₅ give comparable approximations to f ? Explain your observations.