The velocity in ft/s of an object moving along a line is given by v = 3t
Question:
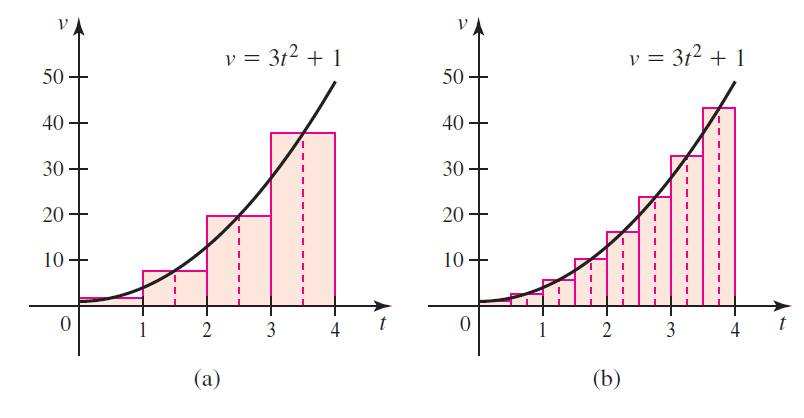
The velocity in ft/s of an object moving along a line is given by v = 3t2 + 1 on the interval 0 ≤ t ≤ 4.
a. Divide the interval [0, 4] into n = 4 subintervals, [0, 1], [1, 2], [2, 3], and [3, 4]. On each subinterval, assume the object moves at a constant velocity equal to v evaluated at the midpoint of the subinterval and use these approximations to estimate the displacement of the object on [0, 4] (see part (a) of the figure).
b. Repeat part (a) for n = 8 subintervals (see part (b) of the figure).
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Calculus Early Transcendentals
ISBN: 978-0321947345
2nd edition
Authors: William L. Briggs, Lyle Cochran, Bernard Gillett
Question Posted:





