Water is drained out of an inverted cone having the same dimensions as the cone depicted in Exercise 29. If the water level drops at 1 ft/min, at what rate is water (in ft³/min) draining from the tank when the water depth is 6 ft?

Data from Exercise 29

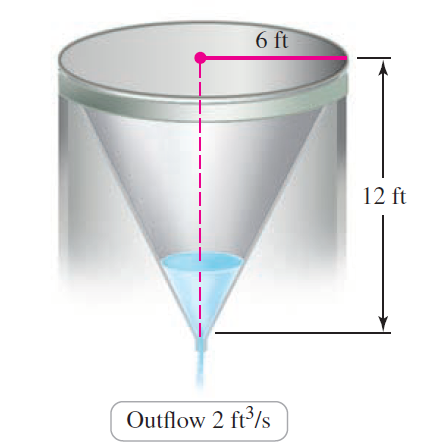
An inverted conical water tank with a height of 12 ft and a radius of 6 ft is drained through a hole in the vertex at a rate of 2 ft³/s (see figure). What is the rate of change of the water depth when the water depth is 3 ft? Use similar triangles.

Transcribed Image Text:

6 ft 12 ft Outflow 2 ft³/s