1. According to the formula for C(n), what is the unit price of the 280th unit, to the nearest thousand dollars?

2. Suppose that instead of using natural logarithms to compute b, we use logarithms with a base of 10 and define b = (log r)/(log 2). Does this change the value of b?

3. All power functions satisfy an equation similar to our functional equation: If ƒ(x) = ax^b, then ƒ(2x) = a(2x)^b = a2^b · x^b = 2^b # ƒ(x). How can you choose a and b to make C(x) = ax^b a solution to the functional equation C(2n) = r · C(n)?

4. Figure 1 on the next page indicates how you could use the integral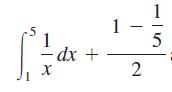

as an estimate for the sum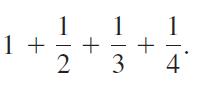

The graph shows the function y = 1/x.

(a) Write a justification for the integral estimate. (Your argument will also justify the integral estimate for the C(n) sum.) Based on your explanation, does the integral expression overestimate the sum?
(b) You know how to integrate the function 1/x. Compute the integral estimate and the actual value. What is the percentage error in the estimate?

-5 1 - dx + X 1- 2 1 5