A fundamental problem in crystallography is the determination of the packing fraction of a crystal lattice, which is the fraction of space occupied by the atoms in the lattice, assuming that the atoms are hard spheres. When the lattice contains exactly two different kinds of atoms, it can be shown that the packing fraction is given by the formula*

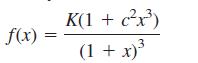
where x = r/R is the ratio of the radii, r and R, of the two kinds of atoms in the lattice, and c and K are positive constants.

a. The function f(x) has exactly one critical number. Find it, and use the second derivative test to classify it as a relative maximum or a relative minimum.

b. The numbers c and K and the domain of f(x) depend on the cell structure in the lattice. For ordinary rock salt: c = 1, K = 2π/3 and the domain is the interval (√2 − 1) ≤ x ≤ 1. Find the largest and smallest values of f(x).

c. Repeat part (b) for -cristobalite, for which

and the domain is 0 ≤ x ≤ 1

d. What can be said about the packing fraction f(x) if r is much larger than R? Answer this question by computing

Transcribed Image Text:

f(x) = K(1 + c²x³) 3 (1 + x)³