Consider the differential equation given by Eq. (12.2). Use a coordinate transformation (zeta=z^{*} P e^{a}), where (a)
Question:
Consider the differential equation given by Eq. (12.2). Use a coordinate transformation \(\zeta=z^{*} P e^{a}\), where \(a\) is some index to be chosen suitably. Show that in the transformed equation there are no free parameters in the convection term and the radial conduction term if the index \(a\) is chosen as one. Also verify that the axial conduction term has a leading coefficient of \(1 / P e\). Hence justify the neglect of the axial conduction term if \(P e\) is, say, greater than 10 .
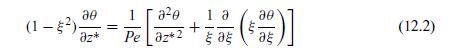
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Advanced Transport Phenomena Analysis Modeling And Computations
ISBN: 9780521762618
1st Edition
Authors: P. A. Ramachandran
Question Posted:





