Show that the Stefan-Maxwell model can be rearranged to define a pseudo-binary diffusivity, (D_{i mathrm{~m}}), for species
Question:
Show that the Stefan-Maxwell model can be rearranged to define a pseudo-binary diffusivity, \(D_{i \mathrm{~m}}\), for species \(i\) in the mixture:
\[\frac{1}{D_{i \mathrm{~m}}}=\frac{\sum_{k=1}^{N}\left(y_{k}-y_{j} N_{k} / N_{j}\right) / D_{j k}}{1-y_{j} \sum_{k}\left[N_{k} / N_{j}\right]}\]
The pseudo-binary diffusivity is seen to be a function of the local mole fraction. A mean average value is often used, and this is treated as a constant. The flux ratios appearing in the above equation are often related by stoichiometry and hence appropriate values can be assigned for heterogeneous reactions occurring at a catalyst surface. Show that for a limiting case in which species 1 diffuses through a "stagnant" species 2 , species 3 , etc. (unimolecular diffusion) the above expression reduces to the Wilke equation, Eq. (9.34). Hence show that the Wilke equation is useful only for a dilute solution of species 1 and also when the fluxes of other species are zero.
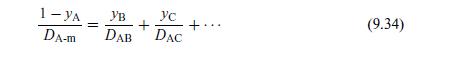
Step by Step Answer:

Advanced Transport Phenomena Analysis Modeling And Computations
ISBN: 9780521762618
1st Edition
Authors: P. A. Ramachandran





