Calculate the wind velocity for the situation shown in Figure 3.44. The plane is known to be moving at 45.0 m/s due north relative to the air mass, while its velocity relative to the ground (its total velocity) is 38.0 m/s in a direction 20.0° west of north.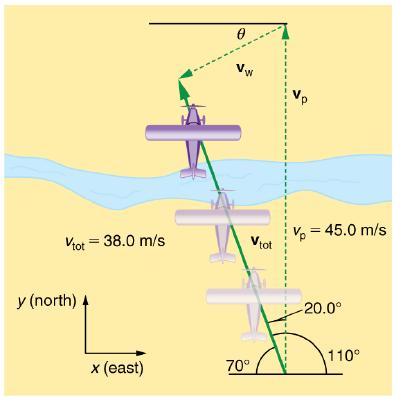

Strategy

In this problem, somewhat different from the previous example, we know the total velocity V_tot and that it is the sum of two other velocities, V_w (the wind) and V_p (the plane relative to the air mass). The quantity V_p is known, and we are asked to find V_w. None of the velocities are perpendicular, but it is possible to find their components along a common set of perpendicular axes. If we can find the components of V_w, then we can combine them to solve for its magnitude and direction. As shown in Figure 3.44, we choose a coordinate system with its x-axis due east and its y-axis due north (parallel to v_p). (You may wish to look back at the discussion of the addition of vectors using perpendicular components in Vector Addition and Subtraction: Analytical Methods.)

Transcribed Image Text:

Vtot = 38.0 m/s y (north) x (east) 0 V. W Vtot 70° 5 Vp = 45.0 m/s -20.0° 110°