Starting with the formula for the moment of inertia of a rod rotated around an axis through one end perpendicular to its length (I = Ml²/3), prove that the moment of inertia of a rod rotated about an axis through its center perpendicular to its length is I = Ml²/12. You will find the graphics in Figure 10.11 useful in visualizing these rotations.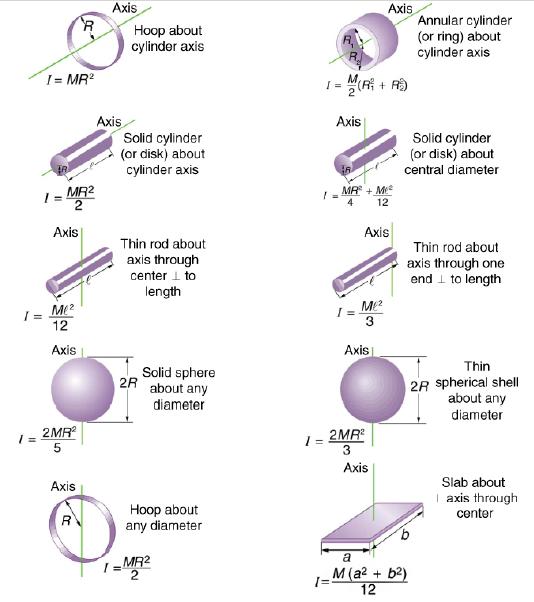

Transcribed Image Text:

1 = 1 = I= MR² 1 = MR² 2 Axis M(² 12 Axis 2MR2 5 R Axis R Axis Axis Hoop about cylinder axis Solid cylinder (or disk) about cylinder axis Thin rod about axis through center ¹ to length Solid sphere 2R about any diameter Hoop about any diameter 1=MR² 2 1 = (= = Axis 1 = MR + Me 4 12 + Axis Me² 3 Axis Axis 2MR2 3 Axis Annular cylinder (or ring) about cylinder axis Solid cylinder (or disk) about central diameter Thin rod about axis through one end to length a M (a² + b²) 12 Thin 2R spherical shell about any diameter Slab about Iaxis through center