A uniform meterstick pivoted at its center, as in Example 8.5, has a \(100-\mathrm{g}\) mass suspended at the \(25.0-\mathrm{cm}\) position.

(a) At what position should a \(75.0-\mathrm{g}\) mass be suspended to put the system in equilibrium?

(b) What mass would have to be suspended at the \(90.0-\mathrm{cm}\) position for the system to be in equilibrium? Example  (8.5): Three masses are suspended from a meterstick as shown in Figure 8.8a. How much mass must be suspended on the right side for the system to be in static equilibrium? (Neglect the mass of the meterstick.)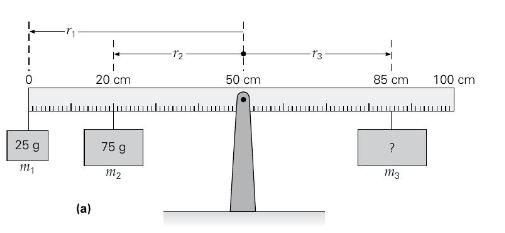 

THINKING IT THROUGH. As the free-body diagram (Figure 8.8b) shows, the translational equilibrium condition will be satisfied with the upward normal force \(\overrightarrow{\mathbf{N}}\) balancing the downward forces caused by the object weights, so long as the stick remains horizontal. But \(\overrightarrow{\mathbf{N}}\) is not known if \(m_{3}\) is unknown, so applying the condition for rotational equilibrium should give the required value of \(m_{3}\). (Note that the lever arms are measured from the pivot point, the center of the meterstick.)13. Telephone and electrical lines are allowed to sag between poles so that the tension will not be too great when something hits or sits on the line.

(a) Is it possible to have the lines perfectly horizontal? Why or why not?

(b) Suppose that a line were stretched almost perfectly horizontally between two poles that are \(30 \mathrm{~m}\) apart. If a \(0.25-\mathrm{kg}\) bird perches on the wire midway between the poles and the wire sags \(1.0 \mathrm{~cm}\), what would be the tension in the wire? (Neglect the mass of the wire.)

Transcribed Image Text:

20 cm 12 25 g (a) 75 g 1112 50 cm 85 cm 100 cm m3