If approximately \(1 \%\) of the electric energy ends up in the \(x\)-ray beam (a typical value), what is the approximate total energy of the \(\mathrm{x}\) rays emitted?

A. \(500 \mathrm{~J}\)

B. \(50 \mathrm{~J}\)

C. \(5 \mathrm{~J}\)

D. \(0.5 \mathrm{~J}\)

\(\mathrm{X}\) rays are a very penetrating form of electromagnetic radiation. \(\mathrm{X}\) rays pass through the soft tissue of the body but are largely stopped by bones and other more dense tissues. This makes x rays very useful for medical and dental purposes, as you know.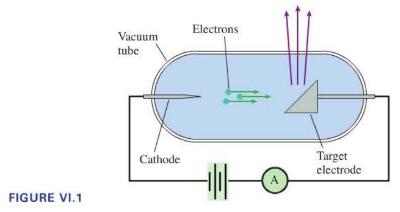

A schematic view of an \(\mathrm{x}\)-ray tube and a driver circuit is given in Figure VI.1. A filament warms the cathode, freeing electrons. These electrons are accelerated by the electric field established by a high-voltage power supply connected between the cathode and a metal target. The electrons accelerate in the direction of the target. The rapid deceleration when they strike the target generates \(\mathrm{x}\) rays. Each electron will emit one or more \(\mathrm{x}\) rays as it comes to rest.
An \(x\)-ray image is essentially a shadow; \(x\) rays darken the film where they pass, but the film stays unexposed, and thus light, where bones or dense tissues block \(x\) rays. An x-ray technician adjusts the quality of an image by adjusting the energy and the intensity of the x-ray beam. This is done by adjusting two parameters: the accelerating voltage and the current through the tube. The accelerating voltage determines the energy of the \(\mathrm{x}\)-ray photons, which can't be greater than the energy of the electrons. The current through the tube determines the number of electrons per second and thus the number of photons emitted. In clinical practice, the exposure is characterized by two values: "kVp" and "mAs." \(\mathrm{kVp}\) is the peak voltage in \(\mathrm{kV}\). The value \(\mathrm{mAs}\) is the product of the current (in \(\mathrm{mA}\) ) and the time (in s) to give a reading in \(\mathrm{mA} \cdot \mathrm{s}\). This is a measure of the total number of electrons that hit the target and thus the number of \(x\) rays emitted.
Typical values for a dental \(x\) ray are a \(k V p\) of 70 (meaning a peak voltage of \(70 \mathrm{kV}\) ) and mAs of 7.5 (which comes from a current of \(10 \mathrm{~mA}\) for \(0.75 \mathrm{~s}\), for a total of \(7.5 \mathrm{mAs}\) ). Assume these values in all of the problems that follow.

Transcribed Image Text:

FIGURE VI.1 Electrons Vacuum tube Cathode A Target electrode