Tracy Wang owns a mortgage banking company. On March 1, she made a commitment to lend a total of $1 million to various homeowners on May 1. The loans are 20-year mortgages carrying a 12 percent coupon, the going interest rate on mortgages at the time, so the mortgages are made at par. Though homeowners would not use the term, we could say that she is buying a forward contract on a mortgage. That is, she agrees on March 1 to give $1 million to her borrowers on May 1 in exchange for principal and interest from them every month for the next 20 years.

Like many mortgage bankers, she has no intention of paying the $1 million out of her own pocket. Rather, she intends to sell the mortgages to an insurance company. The insurance company will actually lend the funds and will receive principal and interest over the next 20 years. Tracy does not currently have an insurance company in mind. She plans to visit the mortgage departments of insurance companies over the next 60 days to sell the mortgages to one or many of them. She sets April 30 as a deadline for making the sale because the borrowers expect the funds on the following day.

Suppose Tracy sells the mortgages to the Acme Insurance Company on April 15. What price will Acme pay for the bonds?

You may think the insurance company will obviously pay $1 million for the loans. However, suppose interest rates have risen above 12 percent by April 15. The insurance company will buy the mortgage at a discount. Suppose the insurance company agrees to pay only $940,000 for the mortgages. Because the mortgage banker agreed to lend a full $1 million to the borrowers, the mortgage banker must come up with the additional $60,000 (= $1,000,00 − 940,000)

out of her own pocket.

Alternatively, suppose interest rates fall below 12 percent by April 15. The mortgages can be sold at a premium under this scenario. If the insurance company buys the mortgages at $1.05 million, the mortgage banker will have made an unexpected profit of $50,000 (= $1,050,000 − 1,000,000) .

Because Tracy is unable to forecast interest rates, this risk is something that she would like to avoid. The risk is summarized in Table 25.3.

Seeing the interest rate risk, students at this point may ask, “What does the mortgage banker get out of this loan to offset her risk bearing?” Tracy wants to sell the mortgages to the insurance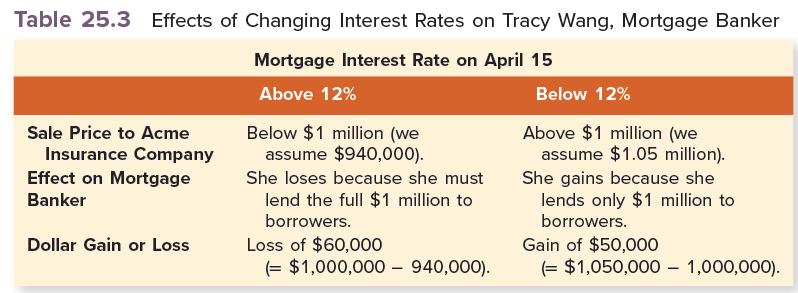

company so that she can get two fees. The first is an origination fee, which is paid to the mortgage banker by the insurance company on April 15—that is, on the date the loan is sold. An industry standard in certain locales is 1 percent of the value of the loan, which is $10,000 ( = .01 × $1 million ) . In addition, Tracy will act as a collection agent for the insurance company. For this service, she will receive a small portion of the outstanding balance of the loan each month. If she is paid .03 percent of the loan each month, she will receive $300 ( = .0003 × $1 million ) in the first month. As the outstanding balance of the loan declines, she will receive less.

Though Tracy will earn profitable fees on the loan, she bears interest rate risk. She loses money if interest rates rise after March 1 and she profits if interest rates fall after March 1. To hedge this risk, she writes June Treasury bond futures contracts on March 1. As with mortgages, Treasury bond futures contracts fall in value if interest rates rise. Because she writes the contract, she makes money on these contracts if they fall in value. Therefore, with an interest rate rise, the loss she endures on the mortgages is offset by the gain she earns in the futures market.

Conversely, Treasury bond futures contracts rise in value if interest rates fall. Because she writes the contracts, she suffers losses on them when rates fall. With an interest rate fall, the profit she makes on the mortgages is offset by the loss she suffers in the futures markets.

The details of this hedging transaction are presented in Table 25.4. The column on the left is labeled “Cash Markets” because the deal in the mortgage market is transacted off an exchange. The column on the right shows the offsetting transactions in the futures market.

Consider the first row. The mortgage banker enters into a forward contract on March 1. She simultaneously writes Treasury bond futures contracts. Ten contracts are written because the deliverable instrument on each contract is $100,000 of Treasury bonds. The total is $ 1 million ( = 10 × $100,000 ) , which is equal to the value of the mortgages. Tracy would prefer to write May Treasury bond futures contracts. Here, Treasury bonds would be delivered on the futures contract during the same month that the loan is funded. Because there is no May T-bond futures contract, Tracy achieves the closest match through a June contract.

If held to maturity, the June contract would obligate the mortgage banker to deliver Treasury bonds in June. Interest rate risk ends in the cash market when the loans are sold. Interest rate risk must be terminated in the futures market at that time. Tracy nets out her position in the futures contracts as soon as the loan is sold to Acme Insurance.

Transcribed Image Text:

Table 25.3 Effects of Changing Interest Rates on Tracy Wang, Mortgage Banker Mortgage Interest Rate on April 15 Above 12% Sale Price to Acme Insurance Company Effect on Mortgage Banker Dollar Gain or Loss Below $1 million (we assume $940,000). She loses because she must lend the full $1 million to borrowers. Loss of $60,000 (= $1,000,000 - 940,000). Below 12% Above $1 million (we assume $1.05 million). She gains because she lends only $1 million to borrowers. Gain of $50,000 (= $1,050,000 - 1,000,000).