Suppose there are four power plants, which use coal, nuclear, wind, and oil, and four cities, Flat

Question:

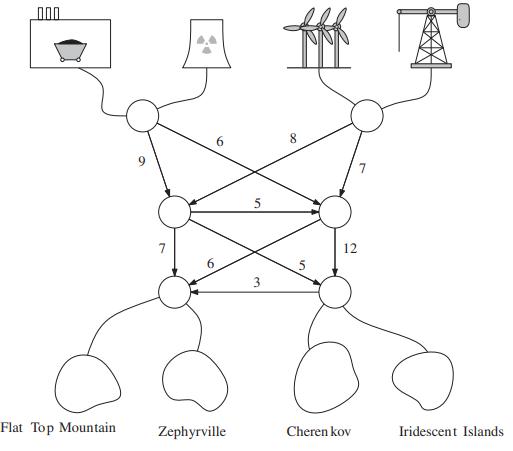
Suppose there are four power plants, which use coal, nuclear, wind, and oil, and four cities, Flat Top Mountain, Zephyrville, Cherenkov, and Iridescent Islands. The power plants and cities are connected by a directed power grid, and each link in the grid has a maximum capacity, as shown in Figure 26.11. Each city demands power with certain constraints. Flat Top Mountain demands 8 units of power, and insists that at least 5 units of this power be from the coal power plant. Zephyrville demands at least 5 units of power and insists that 4 units be generated from wind. Cherenkov demands 8 units of power and insists that 50% of its power be from nuclear power. Iridescent Islands demands at least 6 units of power but wants no more than 2 units of power from oil. Write a linear program to find the maximum flow of power that meets the demands of each city subject to the maximum capacities on the internal links in the power grid.

Figure 26.11

8. 5 7 12 Flat Top Mountain Zephyrville Cheren kov Iridescent Islands