An alternative condition for the convergence of a series of the complex variable (z), namely [S(z)=sum_{i=0}^{infty} f_{i}(z)]

Question:

An alternative condition for the convergence of a series of the complex variable \(z\), namely

\[S(z)=\sum_{i=0}^{\infty} f_{i}(z)\]

is based on the function of \(z\)

\[\begin{equation*}\alpha(z)=\lim _{n \rightarrow \infty}\left|\frac{f_{n+1}(z)}{f_{n}(z)}\right| . \tag{2.261}\end{equation*}\]

The series converges for \(\alpha(z)1\). If \(\alpha(z)=1\) then convergence has to be investigated further.

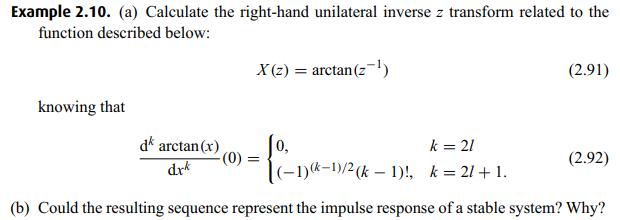
However, for this condition to be applied, no term \(f_{i}(z)\) can be null for all \(z\). In the cases that any \(f_{i}(z)\) is null, one has to create a new sequence of functions \(g_{j}(z)\) composed only of the terms for which \(f_{i}(z) eq 0\) and apply the condition above to this new sequence. With this in mind, solve item (b) of Example 2.10 using the convergence condition above.

image text in transcribed