Consider the block diagram of a satellite control system shown in Fig. P8.6-6. This system is described in Problem 1.4-1. Let \(T=0.1 \mathrm{~s}, K=1, J=0.1\), and \(H_{k}=0.02\). It was shown in Problem 7.5-6 that
\[
G(z)=\frac{z-1}{z} z\left[\frac{10}{s^{3}}ight]=\frac{0.05(z+1)}{(z-1)^{2}}
\]
The frequency response of \({ }_{G(z)}\) is given in Table P7-25. Note that the gain \(H_{k}=0.02\) is not included in this table.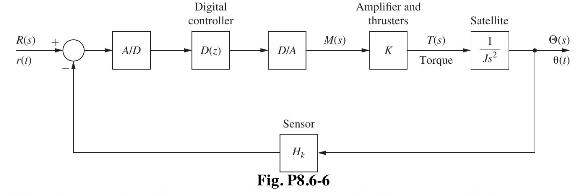
(a) Sketch the complete Nyquist diagram for \(D(z)=1\). Is this system stable?
(b) This system cannot be stabilized by any type of phase-lag controller. Why? Hint: Consider the Nyquist criterion and the Nyquist diagram in part (a).
(c) Design a unity-dc-gain phase-lead controller that yields a system phase margin of \(45^{\circ}\). Hint: Use
\[
\omega_{w 1}=1
\]
(d) Using MATLAB, find the unit step response for the system of part (c). Find the rise time and the percent overshoot for this system.

Problem 1.4-1

Given that (11-21) and (11-22) are valid, derive (11-23).

Transcribed Image Text:

R(s) r(1) A/D Digital controller - D(z) DIA M(s) Sensor 5 Fig. P8.6-6 Amplifier and thrusters K T(s) Torque Satellite I e(s) 8(7)