Consider the system of Fig. P4.10-1. The plant is described by the first-order differential equation [ frac{d
Question:
Consider the system of Fig. P4.10-1. The plant is described by the first-order differential equation
\[
\frac{d y(t)}{d t}+0.04 y(t)=0.2 m(t)
\]
Let \(T=2 \mathrm{~s}\).
(a) Find the system transfer function \(Y(z) / E(z)\).
(b) Draw a discrete simulation diagram, using the results of part (a), and give the state equations for this diagram.
(c) Draw a continuous-time simulation diagram for \(G_{p}(s)\), and given the state equations for this diagram.
(d) Use the state-variable model of part (c) to find a discrete state model for the system. The state vectors of the discrete system and the continuous-time system are to be the same.
(e) Draw a simulation diagram for the discrete state model in part (d).
(f) Use Mason's gain formula to find the transfer function in part (e), which must be the same as that found in part (a). Verify the results in parts (a) and (d) by computer.
Fig. P4.10-1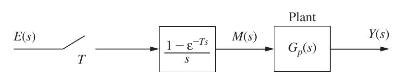
Step by Step Answer:

Digital Control System Analysis And Design
ISBN: 9781292061221
4th Global Edition
Authors: Charles Phillips, H. Nagle, Aranya Chakrabortty




