A quarter car model of an automobile is shown in Figure P4.7. The vehicle is traveling with a velocity \(V\) on a sinusoidal road surface with amplitude \(=0.011 \mathrm{~m}\) and a wavelength of \(5.3 \mathrm{~m}\).

Figure P4.7

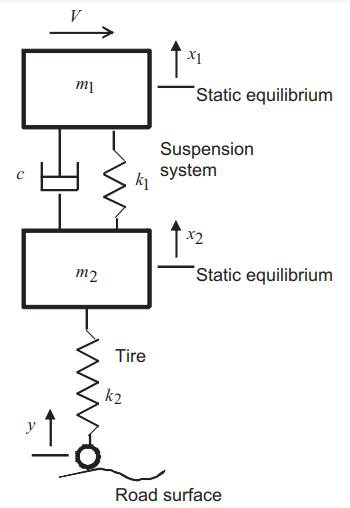
a. Derive the differential equations of motion and obtain mass, stiffness, and damping matrices. Also, obtain the forcing vector.

b. Assume that \(m_{1}=1,010 \mathrm{~kg}, m_{2}=76 \mathrm{~kg}, k_{1}=31,110 \mathrm{~N} / \mathrm{m}, k_{2}=\) \(321,100 \mathrm{~N} / \mathrm{m}\), and \(c=4,980 \mathrm{~N}-\mathrm{sec} / \mathrm{m}\). Determine the damping ratio and the undamped natural frequency for each mode.

c. Compute the amplitudes and the phases of steady-state responses when the velocity \(V=100 \mathrm{~km} / \mathrm{h}\) with and without the damper.

d. Plot amplitudes of both masses as a function of the velocity \(V\) in the presence of a damper.

Transcribed Image Text:

Tu y k1 x1 Static equilibrium Suspension system m2 Tire k2 x2 Static equilibrium Road surface