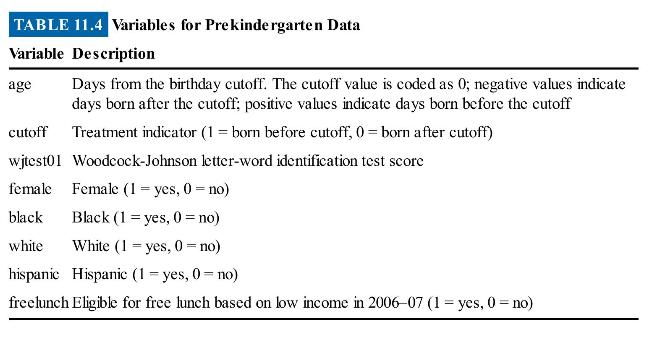
As discussed on page 389, Gormley, Phillips, and Gayer (2008) used \(\mathrm{RD}\) analysis to evaluate the impact of pre-K on test scores in Tulsa. Children born on or before September 1, 2001, were eligible to enroll in the program during the 2005-2006 school year, while children born after this date had to wait to enroll until the 2006-2007 school year. Table 11.4 lists the variables. The pre-K data set covers 1,943 children just beginning the program in 2006-2007 (preschool entrants) and 1,568 children who had just finished the program and began kindergarten in 2006-2007 (preschool alumni).

(a) Why should there be a jump in the dependent variable right at the point where a child's birthday renders him or her eligible to have participated in preschool the previous year (2005-2006) rather than the current year (2006-2007)? Should we see jumps at other points as well?

(b) Assess whether there is a discontinuity at the cutoff for the freelunch status, gender, and race/ethnicity covariates.

(c) Repeat the tests for covariate discontinuities, restricting the sample to a one-month (30-day) window on either side of the cutoff. Do the results change? Why or why not?

(d) Use letter-word identification test score as the dependent variable to estimate a basic RD model controlling for treatment status (born before the cutoff) and the assignment variable (age measured as days from the cutoff). What is the estimated effect of the preschool program on letter-word identification test scores?

(e) Estimate the effect of pre- \(\mathrm{K}\) by using an \(\mathrm{RD}\) specification that allows the relationship to vary on either side of the cutoff. Do the results change? Should we prefer this model? Why or why not?

(f) Add controls for lunch status, gender, and race/ethnicity to the model. Does adding these controls change the results? Why or why not?
(g) Reestimate the model from part (f), limiting the window to one month (30 days) on either side of the cutoff. Do the results change? How do the standard errors in this model compare to those from the model using the full data set?

Transcribed Image Text:

TABLE 11.4 Variables for Prekindergarten Data Variable Description age Days from the birthday cutoff. The cutoff value is coded as 0; negative values indicate days born after the cutoff; positive values indicate days born before the cutoff cutoff Treatment indicator (1 = born before cutoff, 0 = born after cutoff) wjtest01 Woodcock-Johnson letter-word identification test score female Female (1 = yes, 0 = no) black Black (1 yes, 0 = no) white White (1 yes, 0 = no) hispanic Hispanic (1 = yes, 0 = no) freelunch Eligible for free lunch based on low income in 2006-07 (1 = yes, 0 = no)