Consider the cointegrated model (Y_{t}=theta X_{t}+v_{1 t}) and (X_{t}=X_{t-1}+v_{2 t}), where (v_{1 t}) and (v_{2 t}) are
Question:
Consider the cointegrated model \(Y_{t}=\theta X_{t}+v_{1 t}\) and \(X_{t}=X_{t-1}+v_{2 t}\), where \(v_{1 t}\) and \(v_{2 t}\) are mean 0 serially uncorrelated random variables with \(E\left(v_{1 t} v_{2 j}\right)=0\) for all \(t\) and \(j\). Derive the vector error correction model [Equations (17.22) and (17.23)] for \(X\) and \(Y\).
Equations (17.22)
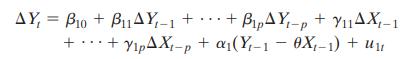
Equations (17.23)
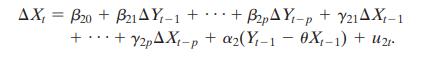
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





