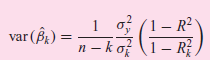For the k-variable regression model, it can be shown that the variance of the kth (k = 2, 3, . . . , K) partial regression coefficient given in Eq. (7.5.6) can also be expressed as

where σ²_y = variance of Y, σ²_k = variance of the kth explanatory variable, R²_k = R² from the regression of X_k on the remaining X variables, and R² = coefficient of determination from the multiple regression, that is, regression of Y on all the X variables.

a. Other things the same, if σ²_k increases, what happens to var (β̂_k)? What are the implications for the multicollinearity problem?

b. What happens to the preceding formula when collinearity is perfect?

c. True or false: €œThe variance of β̂_k decreases as R2 rises, so that the effect of a high R²_k can be offset by a high R².€

(1 R' var (Bt) R}, 1 n - k of k