(left(Y_{i}, X_{1 i}, X_{2 i} ight)) satisfy the assumptions in Key Concept 6.4; in addition, (operatorname{var}left(u_{i} mid...
Question:
\(\left(Y_{i}, X_{1 i}, X_{2 i}\right)\) satisfy the assumptions in Key Concept 6.4; in addition, \(\operatorname{var}\left(u_{i} \mid X_{1 i}, X_{2 i}\right)=4\) and \(\operatorname{var}\left(X_{1 i}\right)=6\). A random sample of size \(n=400\) is drawn from the population.
a. Assume that \(X_{1}\) and \(X_{2}\) are uncorrelated. Compute the variance of \(\hat{\beta}_{1}\). Look at Equation (6.20) in Appendix 6.2.
b. Assume that \(\operatorname{corr}\left(X_{1}, X_{2}\right)=0.5\). Compute the variance of \(\hat{\beta}_{1}\).
c. Comment on the following statements: "When \(X_{1}\) and \(X_{2}\) are correlated, the variance of \(\hat{\beta}_{1}\) is larger than it would be if \(X_{1}\) and \(X_{2}\) were uncorrelated. Thus, if you are interested in \(\beta_{1}\), it is best to leave \(X_{2}\) out of the regression if it is correlated with \(X_{1}\)."
Equation (6.20)
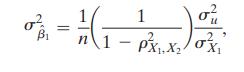
Step by Step Answer:






