Suppose (left(X_{i}, Y_{i} ight)) are i.i.d. with finite fourth moments. Prove that the sample covariance is a
Question:
Suppose \(\left(X_{i}, Y_{i}\right)\) are i.i.d. with finite fourth moments. Prove that the sample covariance is a consistent estimator of the population covariance; that is, \(s_{X Y} \xrightarrow{P} \sigma_{X Y}\), where \(s_{X Y}\) is defined in Equation (3.24).
Equation (3.24)
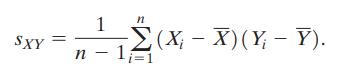
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





